1. What is emissivity?

The [GLOSS]emissivity[/GLOSS] (e) of a real body or surface is defined as the ratio of the radiative flux (E) emitted by the body, to the flux (E_b) from a [GLOSS]black body[/GLOSS] at the same temperature. For a [GLOSS]grey body[/GLOSS] (a gas or surface):

e  =  E/E_b                     (1)

where   e has a value between 0 and 1.

A perfect emitter (black body) has an emissivity of 1.

The emissivity of an opaque object is a function of many factors including:

·          Composition

·          Surface finish or roughness

·          Temperature

·          Wavelength of thermal radiation

·          Angle of emission

Emissivity values for real surfaces are derived by careful measurements in the laboratory and are available in any good heat transfer textbook. However, when selecting data for a given surface, it is important to know the conditions under which the measurements were made, and whether these conditions are similar to those of your surface.

2.Why is it important to know the emissivity?

All surfaces above absolute zero temperature emit radiation.  In most furnaces and combustion chambers, radiation is the dominant mode of heat exchange. The surface emissivity of the load or heat sink (e.g. steam or process tubes) in a furnace directly affects the efficiency of the process. For example, heating a highly reflective material, such as polished stainless steel by thermal radiation is more difficult than heating heavily oxidised steel.

To a lesser extent, the emissivity of the surrounding walls and enclosing surfaces can also affect the overall radiative transfer in a furnace and the thermal efficiency of the process, although the effect is more complex.

Heat losses from the outer casing or walls of combustion equipment include both convective and radiative heat transfer to the ambient surroundings. Use of low emissivity surfaces such as aluminium cladding or paints can help to reduce heat losses.

3. Absorptivity and Reflectivity

The [GLOSS]absorptivity[/GLOSS] (a) of a grey surface is similarly defined as the ratio of energy absorbed by a surface to that absorbed by a black surface exposed to the same incident radiation. Kirchhoff’s Law states that emissivity and absorptivity are equal when thermal equilibrium exists (i.e. when the temperature of the surface (T₁) is equal to the temperature (T₂) of the source of incident radiation). In practice, the emissivity and absorptivity of grey surfaces are equal even when thermal equilibrium does not exist. This is an experimentally observed fact, rather than a universal rule. Hence, if we know the emissivity of a grey surface, then absorptivity is also known. But this rule must not be applied to non-grey real surfaces. 

When radiation falls upon a body, a fraction (a) of it is absorbed, a fraction (r) is reflected, and the remainder (t) is transmitted through the body with:

a  + r + t  = 1                                              (2)

where:  a = absorptivity, r = [GLOSS]reflectivity[/GLOSS], t = [GLOSS]transmissivity[/GLOSS].

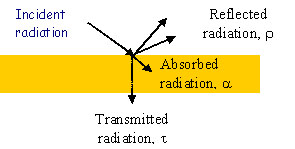
 Figure 1: Reflected, Absorbed and Transmitted Radiation

For most opaque solid materials, all radiation is absorbed within a thin surface layer and transmittance (t) is zero. Hence, 

a  +  r =  1                                          (3)

Since, for a grey surface, absorptivity equals emissivity,

r  =  1  –  e                                              (4)

Thus, for a surface with an emissivity of 0.8, its reflectivity is 0.2 i.e. 20% of incident radiation is reflected from the surface.

4. Measurement of Emissivity

Emissivity is generally determined by measuring the thermal radiation from a surface that is held at a known temperature. The measurement is usually made at a direction normal to the surface, and at a specific wavelength or range of wavelengths depending on the characteristics of the detector. However, various types of emissivity can be quoted in the literature, as follows:

[GLOSS]Spectral emissivity[/GLOSS] – this is the ratio of emission of radiation of wavelength l from the surface, to the radiation from a black body at the same temperature and wavelength.

[GLOSS]Total emissivity[/GLOSS] – this is the ratio of radiation from a surface over all wavelengths, to that from a black body. It is derived using a total radiation pyrometer, or by integrating spectral measurements.

[GLOSS]Normal emissivity[/GLOSS] – this is emissivity measured at a direction normal to the surface.

[GLOSS]Hemispherical emissivity[/GLOSS] – this is emissivity based on the total emission over all directions in a hemisphere about the surface (i.e. over 2p steradians of solid angle).

[GLOSS]Directional emissivity[/GLOSS] – this is emissivity measured at a specific direction from the normal to the surface

The most commonly quoted emissivities in most engineering textbooks are ‘Normal Total’ or ‘Normal Spectral’ emissivities.

5. Emissivity of Gases

Like surfaces, some gases can also absorb and emit infrared radiation. Carbon dioxide and water vapour in fossil fuel combustion products are good examples. A beam of radiation passing through combustion products is attenuated. If the gases are hot, then they also emit thermal radiation.  It is possible to define an emissivity (e_g) for a gas. e_g is however, a strong function of wavelength, and is dependent on the partial pressure of the absorbing gases, their temperature, and more importantly, the beam length for radiation. A small volume of combustion gases with a low partial pressure will have a much lower emissivity than a large volume of gases at high partial pressure. Data on the total emissivity of combustion products from natural gas and oil combustion are available in the literature. These are generally presented graphically, with e_g plotted against T for different values of partial pressure and beam length.

Sources

[1] Robert Siegel & John R.Howell, Thermal Radiation Heat Transfer, 3^rd Edition,

1992, Hemisphere, ISBN 0891162712