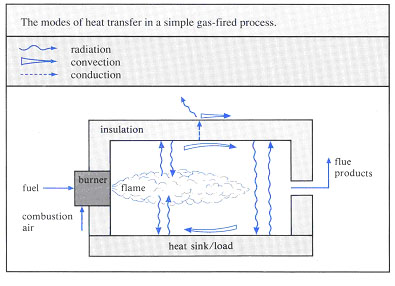
An accurate model of the thermal radiation in furnaces is important for predicting overall thermal efficiency, production rates, quality of the heated product, and pollutant emissions. The prediction of radiative transfer is complex due to the multidimensional and spectral nature of radiation. Thermal radiation exchange is the dominant mode of heat transfer in most furnaces and it depends on many factors including position, local temperature and composition. In order to make progress, a number of techniques have been developed which all introduce simplifying assumptions suited to the particular application or modelling approach.

In a furnace enclosure, the flame and hot combustion gases emit radiation. The radiation is transferred to the load surface via a complex process including:

q       Direct radiation from the flame and combustion products

q       Direct radiation from other surfaces (walls) forming the enclosure

q       Radiation emitted by the gases, that arrives indirectly at the load surface after multiple reflections off the walls and other surfaces in the enclosure. This includes radiation which itself is reflected off the load and re-reflected back to the load.

q       Radiation that is partially reabsorbed (attenuated) by the gases or scattered by particles as it passes through the furnace space. Soot or mineral matter in the flame and combustion products may cause [GLOSS]scattering[/GLOSS].

The problem is further complicated by the fact that combustion gases are not grey. Simple models assume grey behaviour. More complex models include allowance for [GLOSS]non-grey gas[/GLOSS] behaviour.

Furthermore, the combustion atmosphere may not be homogeneous in composition. Where flames extend into the furnace volume, then the composition of radiating components (CO₂, H₂O, carbon and particulate matter) can vary significantly with position as the air and fuel mix, and as combustion progresses.

The different mechanisms of heat transfer in a furnace are illustrated in Fig.1. As well as modelling the internal radiative heat transfer described above, a complete model of a furnace should also take account of convective heat transfer (CF276) and conduction into and through the load and the furnace walls. Complex models may also include prediction of the turbulent fluid flow, mixing and chemical reaction taking place within the furnace.

Fig.1 The heat transfer mechanisms in a typical fuel-fired furnace.

2.    The types of radiation models

Many different approaches to modelling radiation inside furnaces have been published. Broadly, the practical engineering methods can be grouped as follows:

q       The Zone method and related techniques [Hottel, Noble]

q       Flux models [Hottel, Selçuk, Fiveland]

q       Monte Carlo (statistical) techniques [Howell]

q       The Discrete Transfer method (DTM) [Lockwood, Docherty]

Some, such as the flux models, are derived from astro-physics and the modelling of atmospheric radiation. Others, such as the ZONE method were developed specifically as furnace modelling tools. The choice of technique was once critically dependent on the availability of computers with sufficient speed and memory capacity. With modern processing capability this is no longer such a determining issue.

3.    Which method should I use?

The different techniques are described in more detail in CF271, 272, 273 and 274. The following table however, summarises the key features, advantages and disadvantages of these predictive methods.

A Comparison of the Different Methods for Modelling Radiation Transfer in Furnaces